How to calculate the synchronous orbits of the planets?
The synchronous orbit planets is calculated using the law of universal gravitation which boils down to: h=√3(G*M*T2/4π2)-R
h = Height of the artificial satellite
G = Constant of gravitation (6.67*10-11)
M = Mass of the planet
T = Rotational period of the planet
R = Radius of the planet
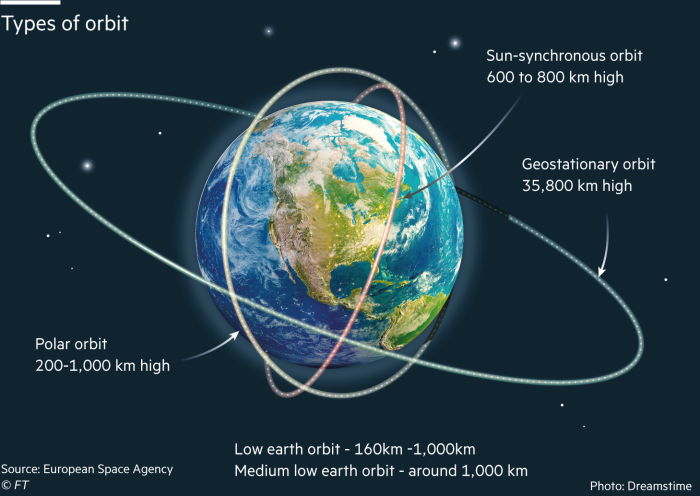
The geosynchronous orbit (synchronous orbit of the Earth) is at an altitude of 35,796 km (≈ 36,000 km) and has a semi-major axis of 42,167 km.
Excel formula used in this table to calculate the altitude of the satellite in synchronous orbit of the planet:
=((((G*M*T^2)/(4*PI()^2))^(1/3))-R*1000)/1000
Excel formula used to calculate the semi-major axis of the synchronous orbit of the planet:
=((((G*M*T^2)/(4*PI()^2))^(1/3)))/1000
- Sun Synchronous Orbit Gif
- Sun Synchronous Orbit Animation
- Sun-synchronous Orbits
- Sun Synchronous Orbit Beta Angle
Sun Synchronous Orbit Gif
The L6 Handbook tells us, “for a nominal sun-synchronous orbit (i.e., one that precesses at a rate of 0.9856°/day or 360°/year), the scene and spacecraft illumination conditions vary throughout the year but repeat on a yearly cycle. A sun-synchronous orbit (SSO), also called a heliosynchronous orbit, is a nearly polar orbit around a planet, in which the satellite passes over any given point of the planet's surface at the same local mean solar time. More technically, it is an orbit arranged so that it precesses through one compl.
Sygic gps maps for windows ce 6. Source: Landsat-6 Programming and Control Handbook
Sun Synchronous Orbit Animation
Sun-synchronous Orbits
Schematic diagram illustrating the geometry of a sun-synchronous orbit for the morning descending node of Landsat.
This schematic appears in the Landsat 6 Programming and Control Handbook that was prepared by Martin Marietta Astro Space in 1993.
Like all of the Landsat satellites, Landsat 6 would have been in a sun-synchronous orbit had its launch been successful.
Sun Synchronous Orbit Beta Angle
The L6 Handbook tells us, “for a nominal sun-synchronous orbit (i.e., one that precesses at a rate of 0.9856°/day or 360°/year), the scene and spacecraft illumination conditions vary throughout the year but repeat on a yearly cycle. The changes during the year occur because apparent Sun motion in the ecliptic plane is non-uniform, whereas the satellite orbit precession is uniform relative to the equatorial plane. The parameter that determines the actual illumination time histories is the orbit o’clock angle. This is the angle between the ascending or descending node and the “mean sun” vector. For a perfect unsynchronized orbit, the orbit o’clock angle remains constant. Furthermore, the Local Mean Sun Time (LMST) of the nodal crossing is the orbit o’clock angle. The relationship is shown in [this figure].”
